How to 'Safely' Gamble
Published: March 14, 2022
A standard game of double-or-nothing has an expected outcome of zero. If you bet $100 you have a 50% chance of winning 100 and a 50% chance of losing 100. The expected outcome is (0.5 * 100 - 0.5 * 100 = 50 - 50 = 0). Over the long run, the average gambler isn't expected to make anything.
If however you had a 60% chance of winning and only a 40% chance of losing then your expected outcome is actually positive. (0.6 * 100 - 0.4 * 100 = 20) These kind of odds would never be offered at a casino since the house would slowly but surely bleed money.
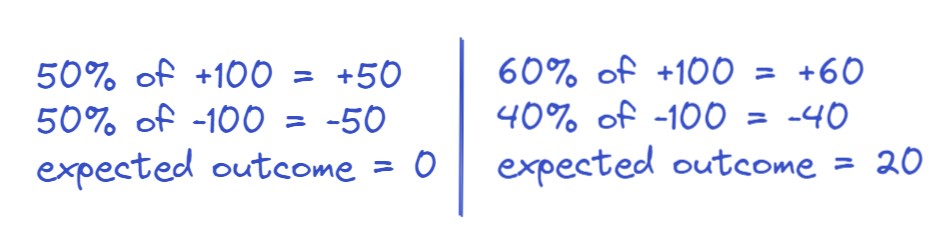
Preview
However if an average person was given this opportunity, they likely wouldn't be able to take advantage of the situation and may even end up with a loss. A 60% chance of winning isn't very high and a 40% loss of everything is a real threat.
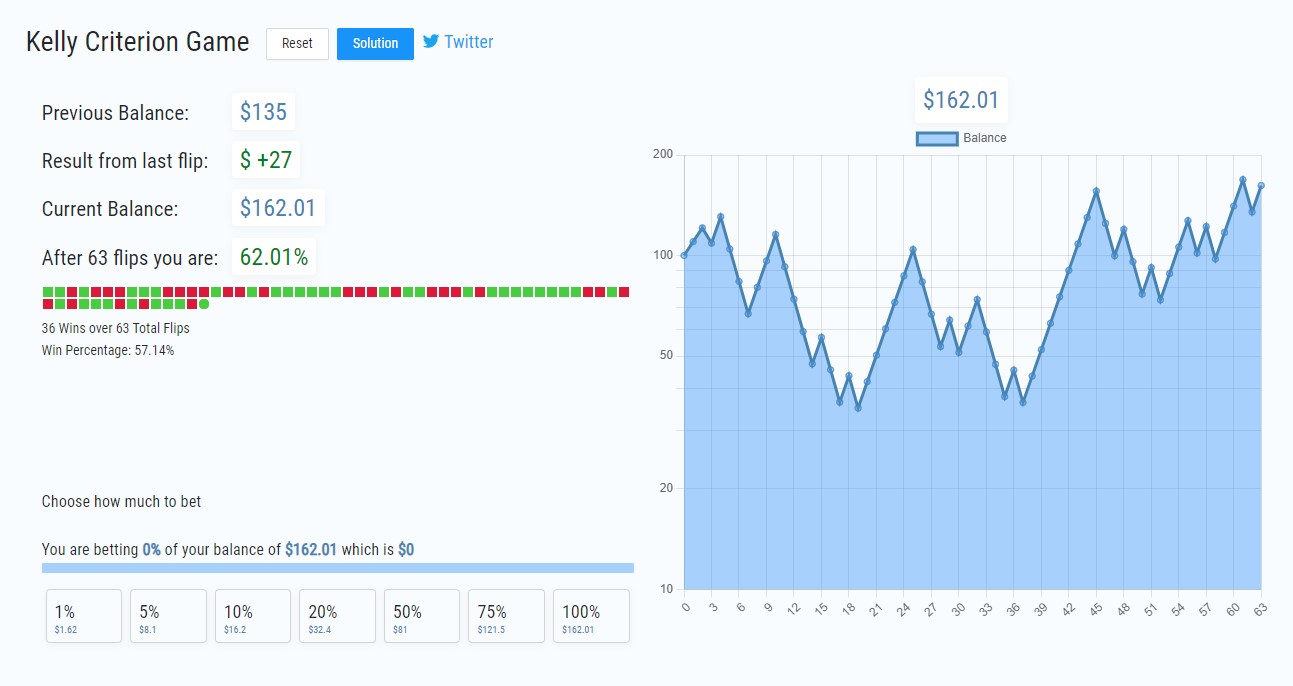
We've developed a game to model this situation. Check it out here. Play around to see if your intuition around how to bet results in more or less money.
Preview
How well did you do? The main concepts the game teaches are the Kelly Criterion and Gambler's Ruin which we'll explore in more detail below.
The Kelly Criterion
The Kelly Criterion is a formula that determine the optimal size for a bet when the expected outcome is known.
If you size too high, you could lose too much money. But if you size too low, you won't take advantage of the opportunity.
Taking advantage of opportunity is important because unlike games, opportunities in reality dry up the more you use them. While you could bet only 1% of your stack each round that would take a very long time and is very unlikely outside of game scenarios.
As Kris Abdelmessih describes in an article on maximizing money:
The bigger you attempt to trade at edgy prices, the more information you leak into the market. You are outsizing the available liquidity by allowing competitors to reverse engineer your thinking.
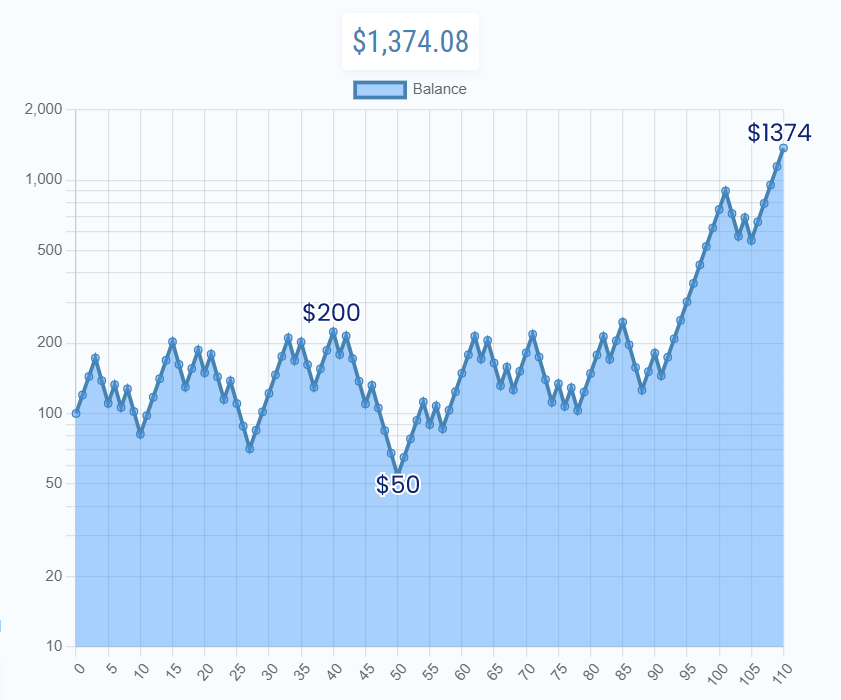
In the game we developed with 60% odds, the Kelly Criterion says that we should bet with 20% of our bank roll each bet. This can feel overly-aggressive. Many would prefer to bet smaller amounts like 1% or 5% to reduce the volatility.
The chart below is a run that uses only 20% kelly betting. Even though we eventually turned $100 to $1374 we had to stomach a $200 to $50 drop. This is a 75% loss on a mathematically guaranteed positive expected outcome game!
Preview
Imagine if you had invested a million dollars and lost 750k. Could you continue to believe in your system? If our software game with a pre-programmed 60% win rate can feel so volatile, imagine how much faith you'd need for your own models where the true win rate is never known.
A safer thing to do is to use the Kelly Criterion as an anchor but apply a "partial kelly" bet.
For example, if you estimate that you have a 60% chance of winning a bet and the Kelly Criterion suggests a bet of 20%, then you could do a "half kelly" bet and only commit 10%. This smooths the volatility as well as the danger that your calculated odds of winning is off.
Below is a run that bets 10% each round. Although the final balance is smaller, the path to get there was less volatile and easier to stomach.
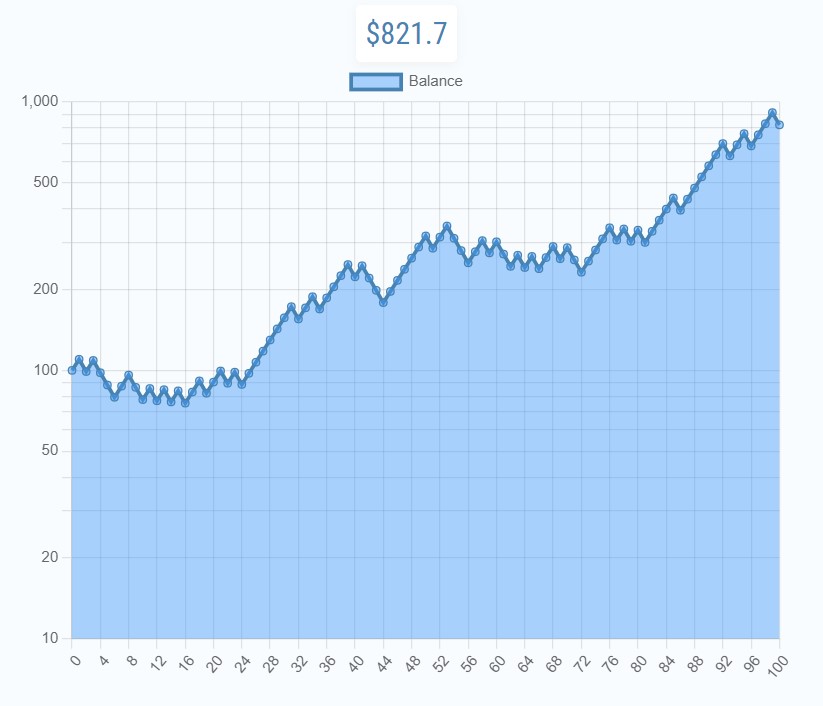
Preview
Gambler's Ruin
Gambler's Ruin is stated:
A persistent gambler who raises his or her bet to a fixed fraction of the gambler's bankroll after a win, but does not reduce it after a loss, will eventually and inevitably go broke, even if each bet has a positive expected value.
Intuitively with our kelly game, if you had bet 100% of your bankroll each round then eventually you'd go broke even though each bet had a positive expected value.
What isn't so obvious is that betting amounts like 50% and 75% also eventually lead to ruin even though some runs can have massive gains. Try it out for yourself. If you continuously bet too high, you will eventually go broke when you encounter an inevitable unlucky streak.
In the run below, $100 turns into $46,000 due to a lucky streak but eventually we are left with less than a dollar.
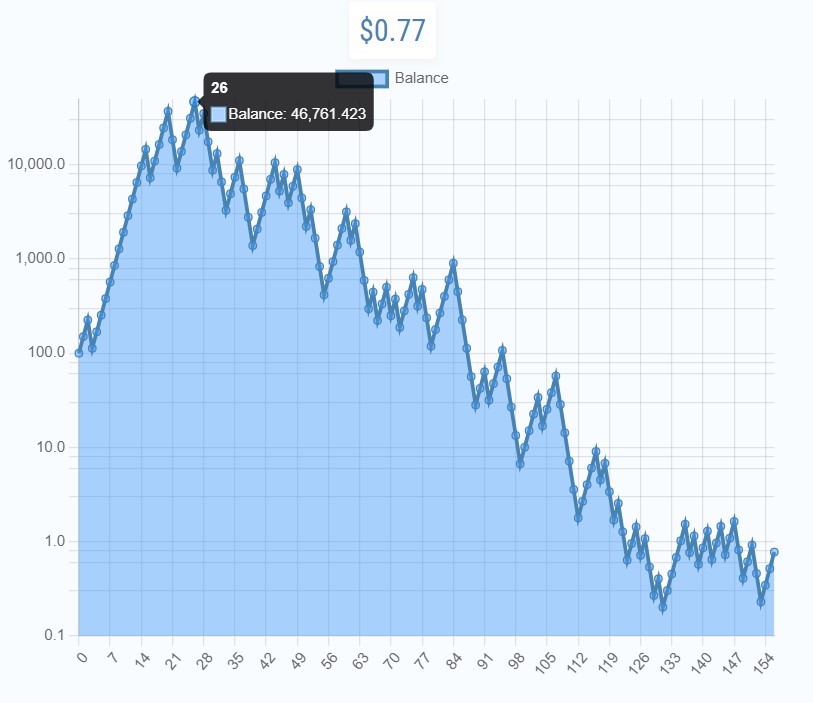
Preview
To emphasize, this is a mathematically guaranteed positive expected outcome game! The effect is exacerbated in real domains like the betting that occurs on /r/wallstreetbets. While the aggressive betting can create grand stories of wealth, they also often end in loss porn.
Value investors like Warren Buffett avoid this type of volatile trading because they have longer time horizons. Their goal is to compound their money over the long run and survive rather than Explode in a burst of glory.
Summary
The Kelly Criterion and Gambler's Ruin show how difficult it is to stick with a strategy in the face of volatility and how easily you can be wiped out if you don't respect it.
The Kelly Criterion is not directly applicable to non-game scenarios since the true probabilities are always hidden. It does however help model how aggressive and defensive you should be with your investing in the face of varying degrees of uncertainty and volatility.
As we have mentioned, stocks that have high P/Es and other accounting ratios typically reach far into the future. While we believe in value investing over the long term, volatile high growth stocks can also be useful ways to grow wealth as long as their position are sized appropriately.